Scaling the Caffeine Buzz
Posted on April 18, 2024 Scale-Up
This post was originally posted as a 3-part series, which are combined below.
Part 1

The science of non-dimensional scaling is universal. Matching the physics of a process at one scale will result in a predictable outcome at another. At Coanda we use dimensional analysis as a starting point for almost all the problems we tackle. In addition to studying complex industrial problems, dimensional analysis can be a used to solve everyday challenges as well. For instance, what if we wanted to make 1,000 cups of coffee in one big volume, to serve a large crowd? We know how to make one really good cup, but how does that help us? How much coffee do we need? Do we need to change the grind size to ensure good contact? What will the dimensions of the puck be? We were asked to tackle this problem by Dr. William McCaffrey (Chemical and Materials Engineering, University of Alberta, Edmonton), and to present the results during the course he had developed on the engineering science underpinning coffee making. Over a series of three posts, we will show how scaling by dimensional analysis can point us to a solution. The first will frame the problem. Next, we will develop the scaling rules, and the final post will put it all together.
To start, lets consider the basics of what we are trying to achieve. The process of making coffee is a diffusion problem. Hot water flows across a packed bed of coffee, and the awesomeness in the coffee beans is extracted from the solid grinds into the water. Since the flavour compounds are depleted from the beans as water flows over them, the first drop of coffee liquid into the cup is more concentrated than the last one. We need to make sure that when all the liquid that has contacted the beans is mixed together it has just the right amount of extracted material. It is this final concentration that we are trying to replicate. So at the highest level, we are interested in scaling a diffusional process, where the integral value of the amount of soluble compounds transferred from the solid beans to the water, per volume is maintained. In a future post we will set up the scaling rules on how to achieve this – stay tuned.
Part 2
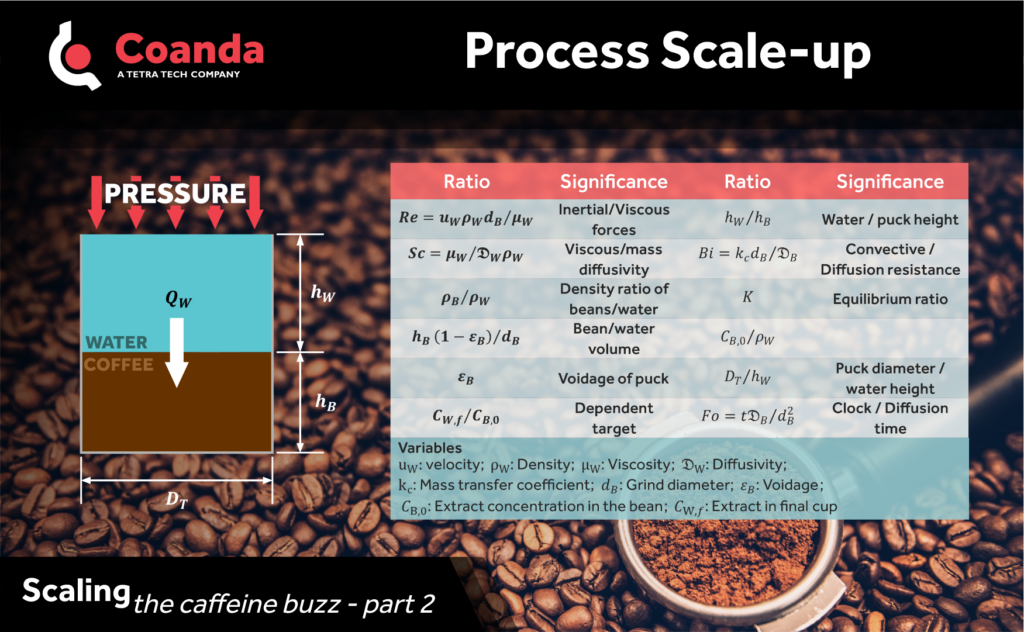
So, now we know how to make one really good cup of coffee, we want to design an espresso maker to make 1,000 cups in one BIG shot and taste just as good! First, we need to list the things that matter to the coffee making process that are under our control. We can group this list into things about the water, the coffee puck, and the espresso maker. Next, we arrange these variables into groupings that represent ratios of forces, velocities, geometry, and physical properties. Since these groupings are ratios of the same type of quantity (for instance the height and diameter of the coffee puck), each ratio has no dimensions.
The number of such groupings that we can form is defined by the mathematics underpinning dimensional analysis, but the specific list we end up with is at our discretion. We use the experience and insight from related problems that others have solved to guide us. For instance, it has been well established that the ratio of the inertial to viscous forces in the flowing liquid is important in mass transport, and so we include this ratio in the final list. Similarly, a physical meaning can be extracted from all ratios in the list, and the process of extracting this insight is an important (and sometimes difficult!) part of the scaling process. For instance, with a bit of work we can identify the grouping hB(1-eB)/dB as the total volume of beans that a volume of water passes through.
With the independent list defined, we now select the dependent variable of interest. In our last post, we suggested the taste is dictated by the concentration of bean extract in the final liquid. In non-dimensional form we reference this concentration to that in the original bean.
We have now set up the scaling framework. If all the non-dimensional groups are matched exactly to their small-scale values, then the final concentration of flavour compounds in the cup will be the same as that in the single cup preparation that we have perfected. How we do that, and some of the issues that can arise will be discussed in our final post in this series.
Part 3
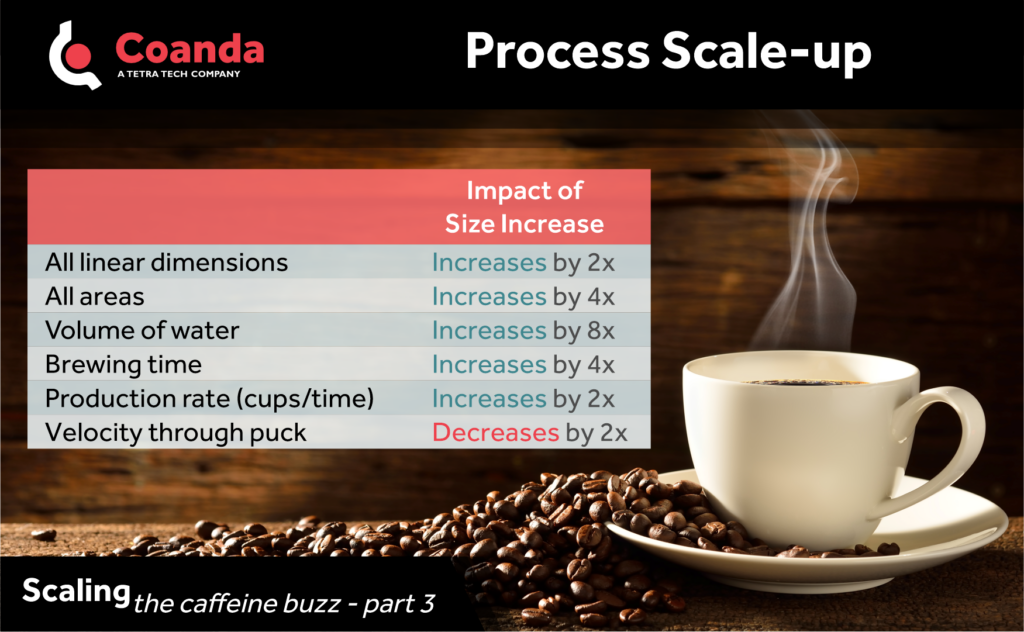
In the last post, we developed the scaling relationships for replicating a single perfect cup of coffee at a large scale. By matching all the independent ratios, the dependent ratio of interest (ie. the quality of the final liquid) should be replicated. To start, we need to ensure that the geometry is scaled correctly. Let’s pick a scaling factor of two and see what impact that has. We see that doubling the size of our machine, we produce 8 times the volume of coffee, and it takes 4 times longer.
Now we encounter the elephant in the room. Since we are using the same fluids and beans as the single-cup case, the physical properties will be identical at the large scale. As we have changed the geometry, we need to ensure that the other non-dimensional variables in the scaling framework are not mismatched by this change. We note that:
-All ratios based solely on physical properties remain constant.
-The change in velocity and grind size exactly offset, so the inertial/viscous forces remain constant.
The ratio of convection/diffusion resistance is tricker, as it contains the mass transfer coefficient kc. Here we recognize the well-known relationship relating the non-dimensional form of the mass transfer coefficient kcdp/Dw=fn(Re,Sc). Since Re and Sc are exactly matched, then kcdp/Dw will also remain constant, and kc will decrease by a factor of 2. This decrease exactly offsets the increase in grind size, and the ratio of convection/diffusion resistance is identical in the scaled coffee maker.
With the scaling framework validated, it follows that that to produce 1,000 cups of coffee, we need to increase all dimensions by a factor of 10, and the time to brew our mega-batch will increase by a factor of 100. This will mean that the batch will have to be kept warm for 50 minutes or so. It cannot be served before the batch is complete, since the last and first drops collected contain very different concentrations of extract.
This simplified example introduces the basic utility of non-dimensional scaling, an approach that can be applied to the most complex of engineering problems!


