News + Publications

A large number of industrial and geophysical fluids exhibit a yield stress, where a certain critical stress must be exceeded before the material will start flowing, below this critical stress the material behaves as a solid. Fluids that can have a yield stress include mud, cement, lava, fine particle suspensions, foams, molten plastics and metals, processed foods, and many more. At Coanda we have a wide range of experience working with these non-Newtonian fluids.

For one such project, we needed to model the slow (i.e., low Reynolds number) deposition of layers of a yield stress fluid where the yield stress of the fluid increased with time.

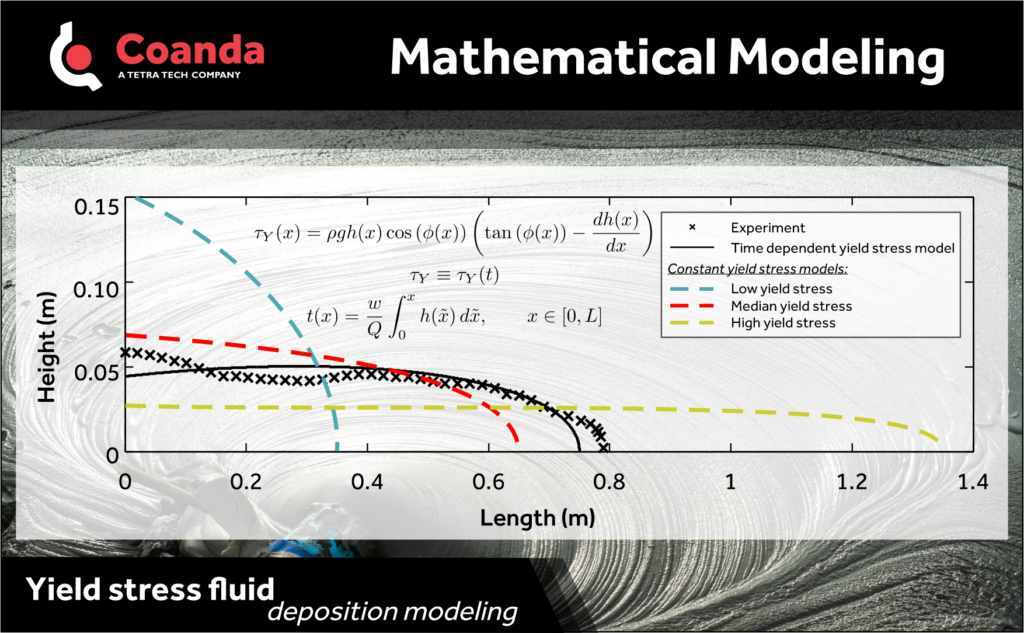
A well-developed shallow layer theory exits in the literature for the slow flow of yield stress fluids over rigid surfaces—for fluids with a constant yield stress. Initially, this theory was being used to estimate the deposition behavior, using some representative constant yield stress value. However, it was unclear how significant the effect of the changing yield stress might be.

To investigate this, we leveraged the existing academic work and modified the shallow layer theory equations to include a time-dependent rheology. It was found that in some scenarios, regardless of how you chose a representative yield stress, using a constant yield stress to model the deposition always displayed some discrepancies with the predicted deposit shape. In these cases, the time-dependent yield stress model was able to better model the overall shape of the deposit.